Video: Nodes and antinodes of vibration
The vibration one observes in a guitar string, once it has been plucked, is the sum of an infinity of stationary waves called harmonics, or modes of vibration.
The tension, adjusted by the tuning pegs, and the length of the string, impose upon the waves that are propagated certain conditions of frequency and wavelength.
The two extremities of the string define limiting conditions, which impose zero displacement at these points, at all times.
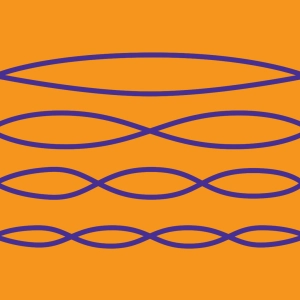
This simulation enables us to observe the first four harmonics separately, which is not possible in reality.
(…)
The stationary wave that has the greatest wavelength, and is in accord with the limiting conditions, is the first harmonic.
It is also called the fundamental.
It has two nodes of vibration at the two extremities and a single antinode in the middle, which gives it this very characteristic bulging envelope shape.
(…)
Its resonant frequency , noted as f0 depends on the length of the string: the shorter the string, the higher the frequency is, and the higher the pitch of the sound is.
(…)
A harmonic of rank “n” is a stationary wave with a frequency n times the frequency of the fundamental.
(…)
However, even if the series of harmonics is infinite, their respective amplitudes decrease very rapidly, and only the first harmonics, among which is the fundamental, are perceived by our ears.
