Video: Modes of vibration (harmonics)
Video: Modes of vibration (harmonics)
For a string that is fixed at both ends, and for which we know the tension and the length, we know how to determine the values of the resonant frequencies of waves that are able to be maintained on that string.
Each of these waves is a mode of vibration.
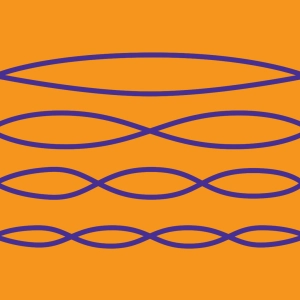
There are an infinite number of these. Here are the first four of them.
Note that the physicist, like the musician, calls these modes harmonics.
But when we pluck a guitar string, precisely which of these modes do we observe? (…)
Actually, all of them!
The motion of the string is in fact derived from the superposition of all of the modes.
The first mode, or first harmonic, is called the fundamental.
Its envelope, with a single antinode at the center, is characteristic of this mode.
Its frequency, F0, is called the fundamental frequency and it depends, among other things, on the length L of the string.
The shorter the string, the higher the frequency, and the higher the pitch of the sound. (…)
The second harmonic vibrates at a frequency that is double that of the fundamental.
It has two antinodes and three nodes of vibration. (…)
More generally, the harmonic of rank “n” oscillates with a frequency “n” times that of the fundamental. (…)
Let’s remember that the higher we go in the ranks of the harmonics, the higher the frequency is, but the lower the amplitude becomes.
It is therefore the fundamental note that predominates in our auditory perception.

Discover EduMedia for free
The interactive encyclopedia that brings science and math to life in the classroom.
Over 1,000 resources