 Video: The harmonics of a note
Video: The harmonics of a note
To produce sound, a musical instrument must perform two functions :
- Vibrate
- and emit
For most instruments, these two functions are inseparable.
Thus, the skin of a drum that vibrates is also the source of the sound waves that escape from it.
(…)
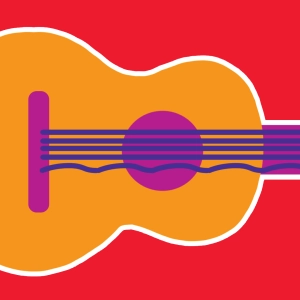
The vibrating strings of a guitar or a piano are not large enough to agitate the air, and so emit a sound. We therefore add a resonance box which, via its larger surface and specially adapted shape, acts as an amplifier.
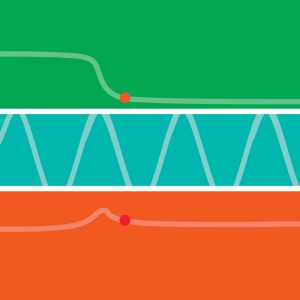
When we play the note C (…) on a piano for example, here, amplified and slowed down, is the shape of the vibration that appears on the string.
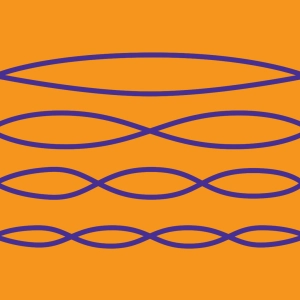
This vibration, which appears complex, is actually the superposition of an infinite number of vibrations called the harmonics.
(…)
The first is called the fundamental. It vibrates at frequency f0.
The harmonics that follow have frequencies that are multiples of f0 and each one of them corresponds to a note.
Put another way, when we play the note C we make other notes resonate.
(…)
The second harmonic vibrates at a frequency that is double that of the fundamental.
It’s a C one octave higher.
The third harmonic turns out to be G, the fifth of C (…)The harmonic of rank 5 is E, the third of C (…) , and still more notes follow as we climb higher in the harmonics.
That which we hear in playing C is the sound produced by the superposition of all of these vibrations.
The relative amplitudes of the harmonics characterize the timbre of an instrument.
(…)
What we just saw in the case of a piano string also applies to the column of air in a saxophone.
When the same note is played on different instruments, the harmonics will have the same frequencies, corresponding to the same notes, but the relative amplitudes will be different.
(…)
Note that the third and the fifth, associated with the tonic C, make up what musicians call the tonic chord.
(…)
It sounds very harmonious and that can be given a physical explanation by the fact that the notes E and G that are played reinforce the harmonics E and G already present in the note C.

Discover EduMedia for free
The interactive encyclopedia that brings science and math to life in the classroom.
Over 1,000 resources